Case 4: Price competition (computerised)

One of our favorite computerised experiments is on Bertrand competition (available on both Veconlab and FEELE). We have had success running this experiment with students from sixth form level up to corporate executives. Students act as firms in a market. Each period in time, they choose prices. The customers (played by the computer) go to the firm with the lowest price (in the event of a tie, the demand is split equally). Each firm has constant marginal cost and, given the demand of the consumers, the Nash equilibrium is for firms to charge a price equal to their marginal cost, leading to zero profits (see Kaplan and Wettstein, 2000).

You can see the results of the experiment in the figure below. These results are typical. With two firms in a repeated situation, the prediction of perfect competition fails. Even without explicit communication, firms can collude. To quote an anonymous student:

‘I learnt that collusion can take place in a competitive market even without any actual meeting taking place between the two parties.’

This changes quite drastically for a larger number of firms and random matching. Here, the competition is fierce and the profits are driven out of the market. To quote another student:

‘Some people are undercutting bastards!!! Seriously though, it was interesting to see how the theory is shown in practice.’

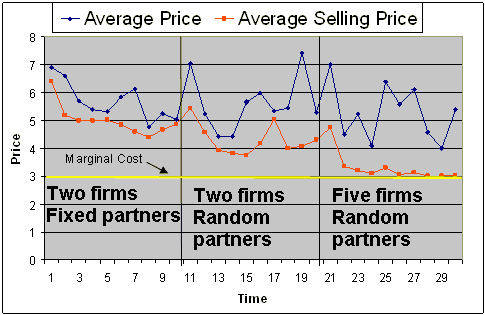
It is especially important in this experiment to display the selling price in addition to the average price chosen since that indicates the profits in the market. Only by seeing the selling price can one clearly see the strength of the equilibrium prediction.

Bertrand competition with complements

A lecture on industrial organisation will discuss the advantages and disadvantages of different market structures. A counter-intuitive concept is that more competition is not always better. Duopoly may be worse than monopoly. This is the case when a monopoly sells two complementary goods and is then split into two firms to sell each good separately. The theoretical analysis shows that consumers pay a higher price for a pair of commodities after the split. In a crude analogy, being robbed twice is worse than being robbed once for the consumer. The analysis is clearly relevant for competition policy: for instance, the decision on whether to split Microsoft up into two separate companies, one that sells the Windows operating system and one that sells Microsoft Office (Excel, Word, etc.) Krugman (2000) argues just this in his column entitled ‘Microsoft: What Next?’ In agreement with the economic analysis, the US government agencies decided against such a split.

To convey this concept, FEELE provides a computerised experiment based upon a similar hand-run experiment by Beckman (2003).

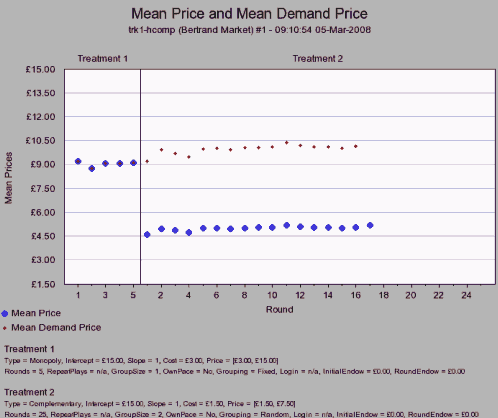
Looking at the following graph of results, we started students in a monopoly situation facing a demand of 15p and a constant marginal cost of 3p. The profit maximising price is 9p. Students found this price fairly rapidly. When we broke up the company into two separate companies producing complements and competing in a duopoly, there was a clear increase in the price to over 10p (the equilibrium price is 11p).

It is of particular teaching and learning value that the model is just a seemingly minor variation of the standard model of price competition which we use in microeconomics. (The standard model uses perfect substitutes instead of perfect complements.) For the standard model one observes sharp cut-throat competition which erodes profit possibilities: a completely opposite result.

One can run a combination of both Bertrand games against former subjects with the following:

http://www.projects.ex.ac.uk/feele/feele_experiments/subject_access.php?quick=bertrand